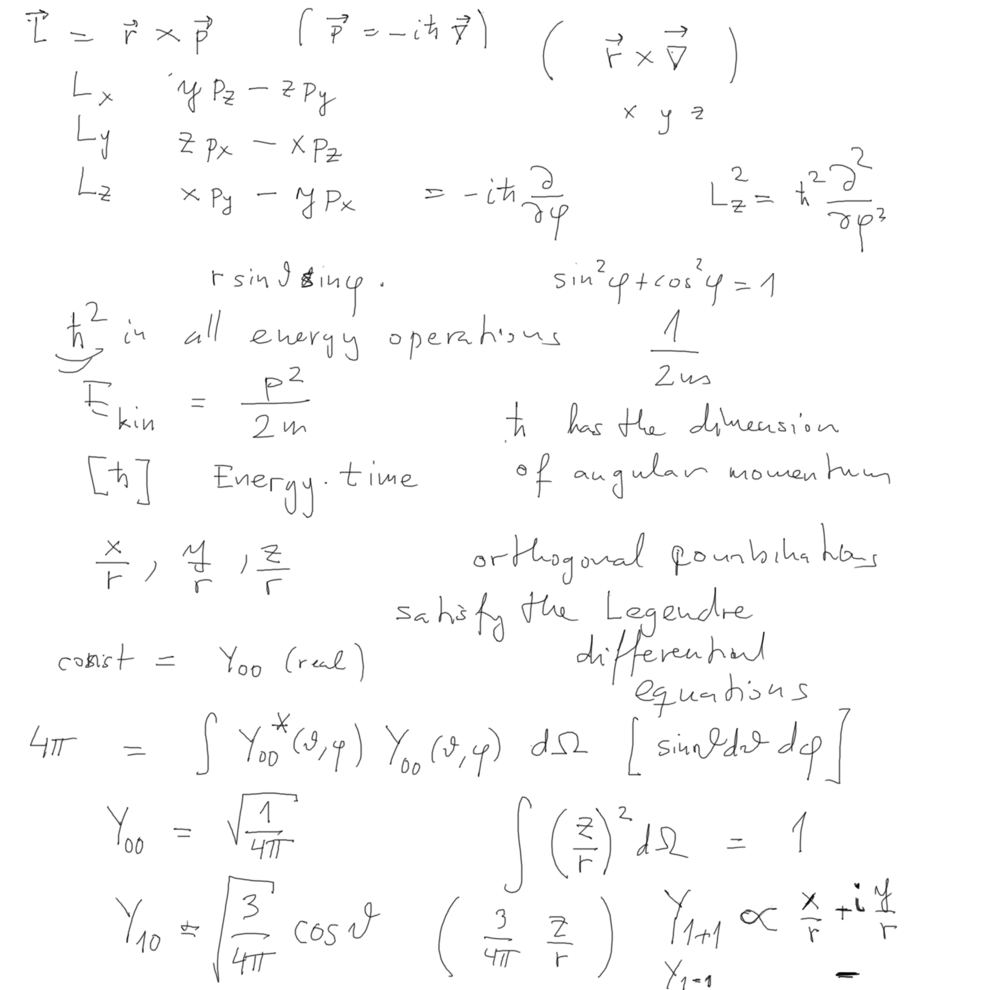Basic Knowledge - Hydrogen
Atom
Here we continue the introduction, mainly directed to basic knowledge
about the hydrogen-like
atomic
systems.
Extra pages on graphics Graphical
study
of
Hydrogen
Bound
States
(MATLAB)
These are mentioned in most introductory courses of Modern Physics,
Quantum mechanics etc
Those are:
Schrödinger equation for Coulomb potential
Separation of radial and angular parts;
Spherical harmonics
Eigenvalues; Bound states of hydrogen
Energy levels; Spectra of hydrogen
Selection rules
Most of these basic topics are covered for example in these
LINKS:
Hydrogen atom wavefunctions http://en.wikipedia.org/wiki/Hydrogen_atom#Wavefunction
Laplace operator http://en.wikipedia.org/wiki/Laplace_operator#Three_dimensions
Legendre Polynomials http://en.wikipedia.org/wiki/Legendre_polynomials
Spherical harmonics http://en.wikipedia.org/wiki/Spherical_harmonics
Table of them http://en.wikipedia.org/wiki/Table_of_spherical_harmonics
List of Radial functions: http://en.citizendium.org/wiki/hydrogen-like_atom#List_of_radial_functions
see also the whole article http://en.citizendium.org/wiki/hydrogen-like_atom
- better than wikipedia
Schrödinger equation for
radial potential (central potential, spherically symmetric, isotropic
.......)
and thus for the point charge Coulomb potential.
Separation of Variables, Radial
and Angular; Angular Momentum operator - see also below
xcf_b011.png

xcf_b011.png
Separation r and angles; Further separation - theta and phi;
For phi - instead of boundary condition - the periodicity over 360
degrees is used
Angular Momentum operator (Squared L) - Angular part
-> Legendre Polynomials http://en.wikipedia.org/wiki/Legendre_polynomials
xcf_b020.png

xcf_b020.png
Angular Momentum operator --> angular
variables only - see the dimensional analysis
Angular Momentum has the dimension of Action - Planck's constant.
Angular momentum quantized - in units of h-bar
xcf_b030.png
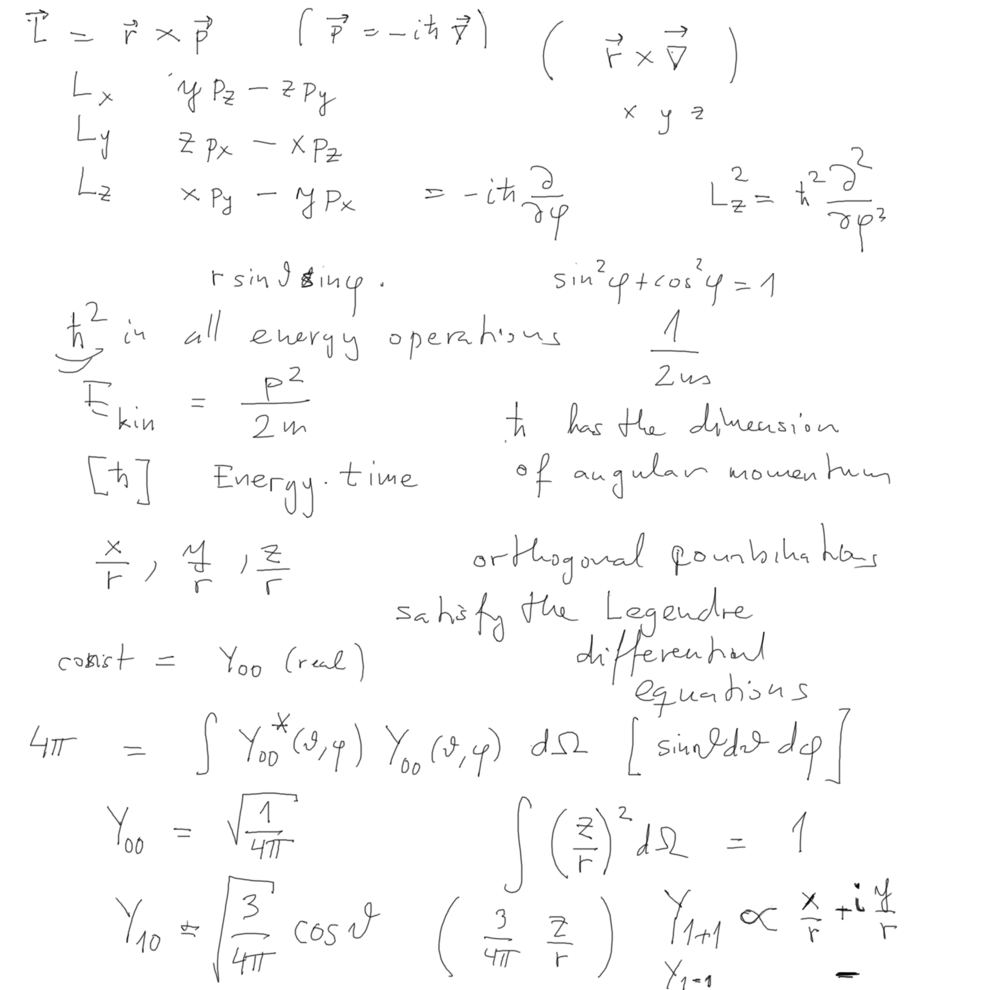
xcf_b030.png
Spherical harmonics are (also) orthogonalized polynomials of
x/r y/r and
z/r - see above
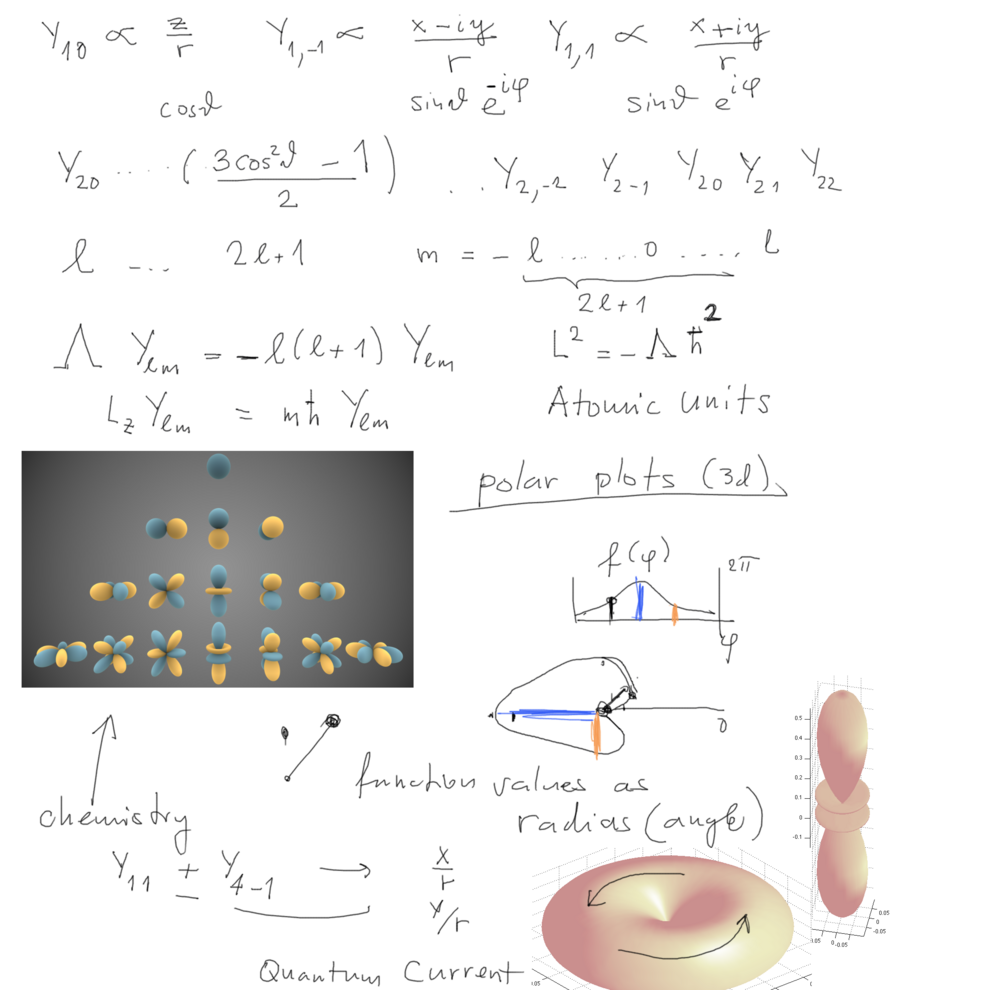
Spherical harmonics, polar plots, Popular Culture, Wikipedia
Note:
wikipedia has chemistry - prefered REAL-valued spherical harmonics as
illustrations
xcf_b040.png
xcf_b040.png
See the complex valued Ylm - extra pages on graphics
Graphical
study
of
Hydrogen
Bound
States
(MATLAB)
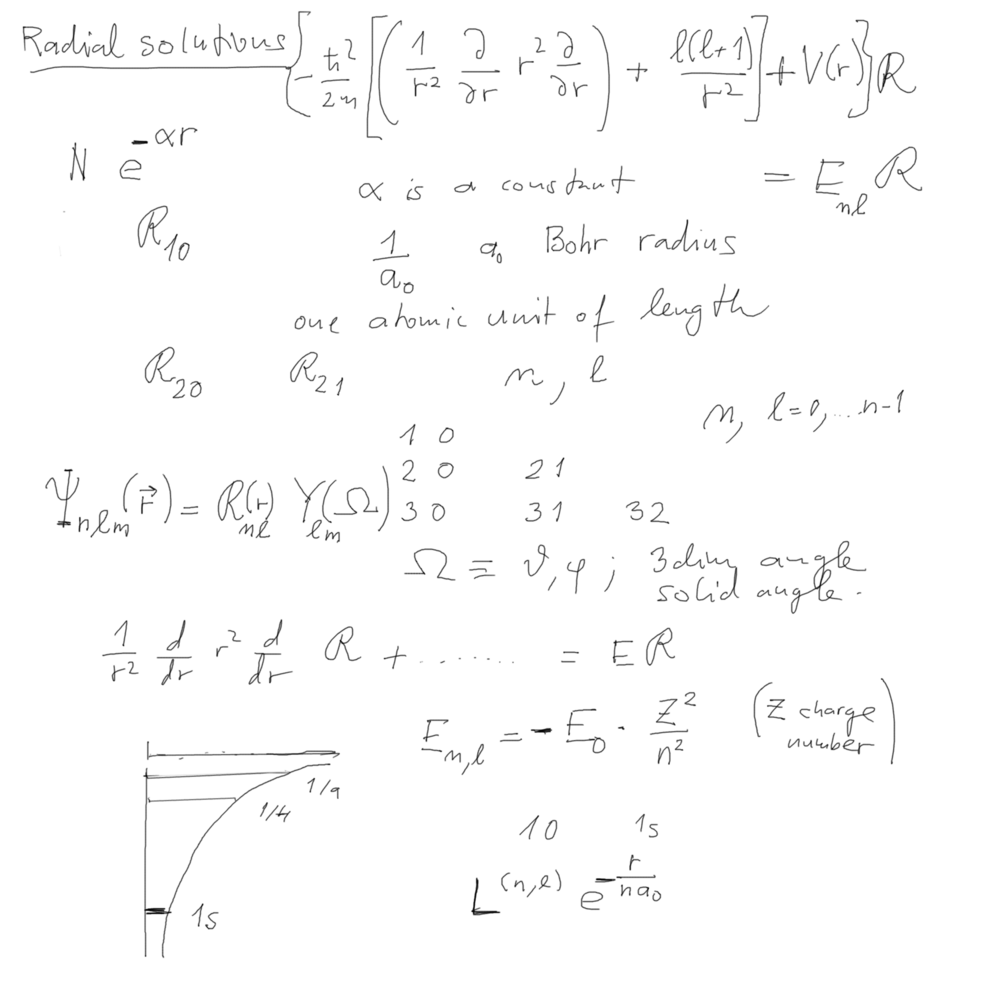
Schrödinger equation for
radial potential (central potential, spherically symmetric, isotropic
.......)
and thus for the point charge Coulomb potential.
Separation of Variables, Radial
PART
xcf_b050.png
xcf_b050.png
Solutions are called Laguerre Polynomials multiplied by exponential
List of Radial functions:
http://en.citizendium.org/wiki/hydrogen-like_atom#List_of_radial_functions
see also the whole article http://en.citizendium.org/wiki/hydrogen-like_atom
- better than wikipedia
Energies: 13.6( Z2 / n2 ) eV
(electronVolts)
Ask
Google: 1
electron volt = 1.60217657 × 10-19 joules
Build energy levels - combine to spectra
Relation of wavelengths - energies
xcf_b060.png
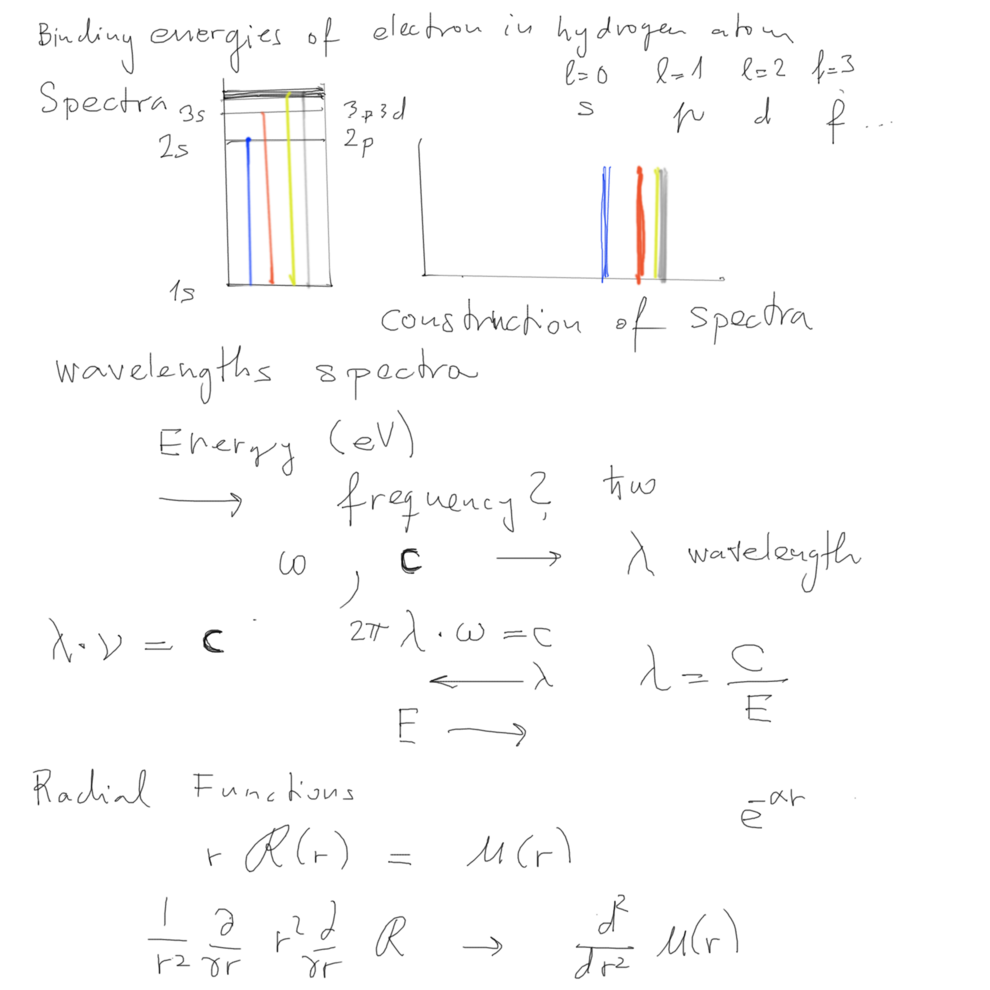
xcf_b060.png
Psi_hydrogen_citizendum.png

Psi_hydrogen_citizendum.png
Relation of wavelengths - energies - program lines for
Octave or MATLAB