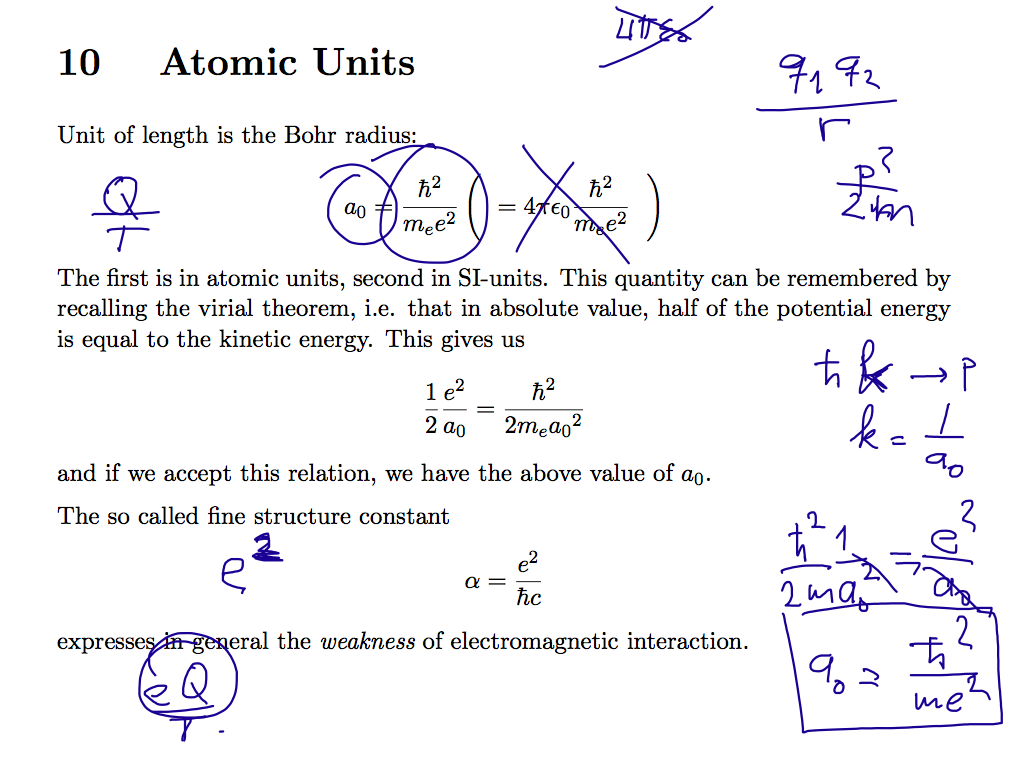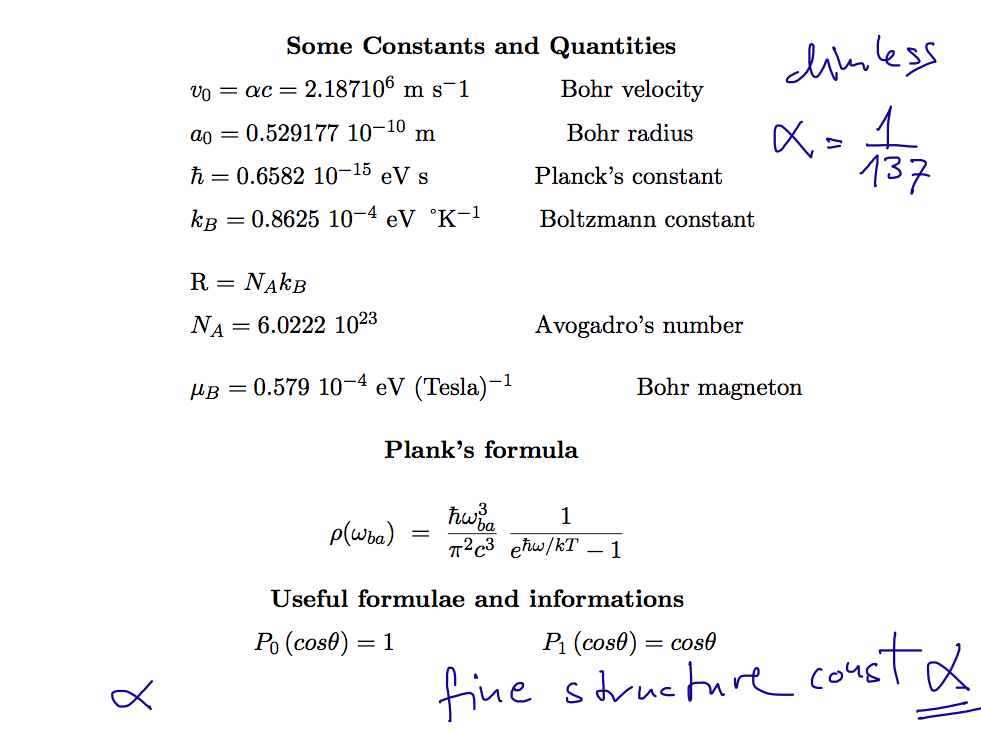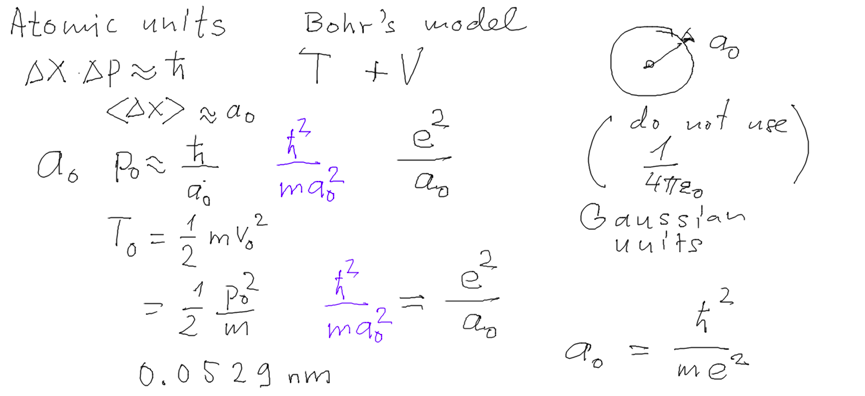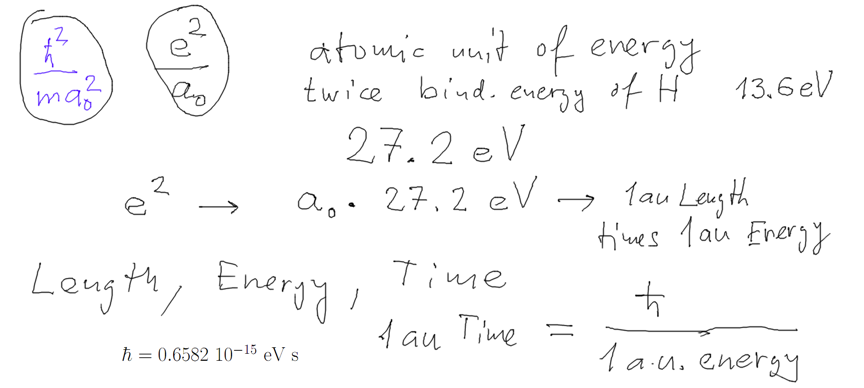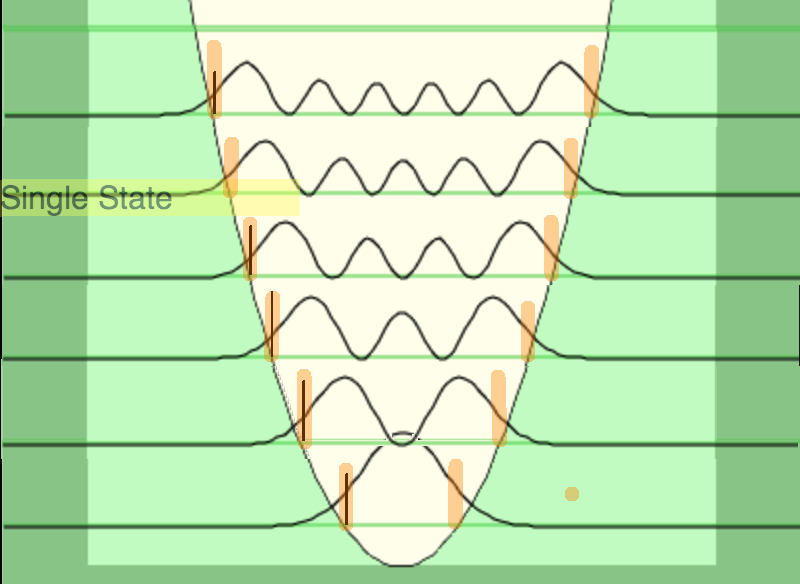
02_turning_pointz_harmonic_oscillator.png
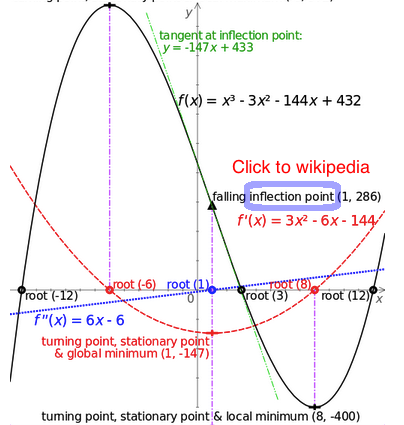
Inflection point at Wikipedia

02_turning_pointz_harmonic_oscillator.png
 02_turning_pointz_harmonic_oscillator.png |
02_wiki_INFLECTION_POINT.png Inflection point at Wikipedia |

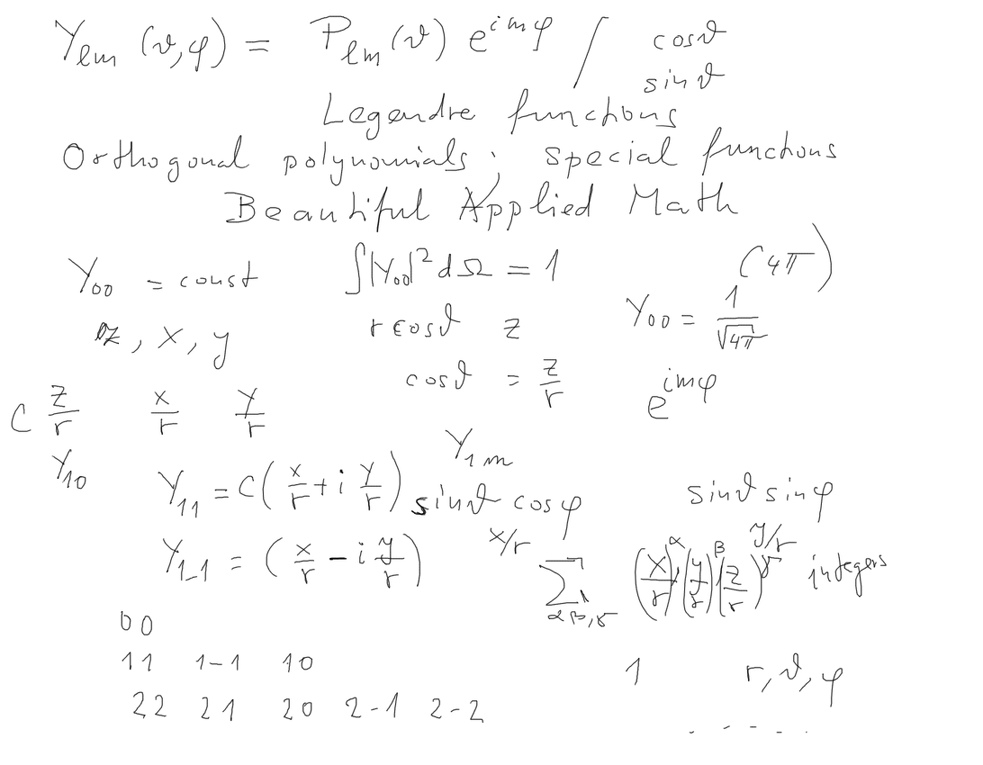
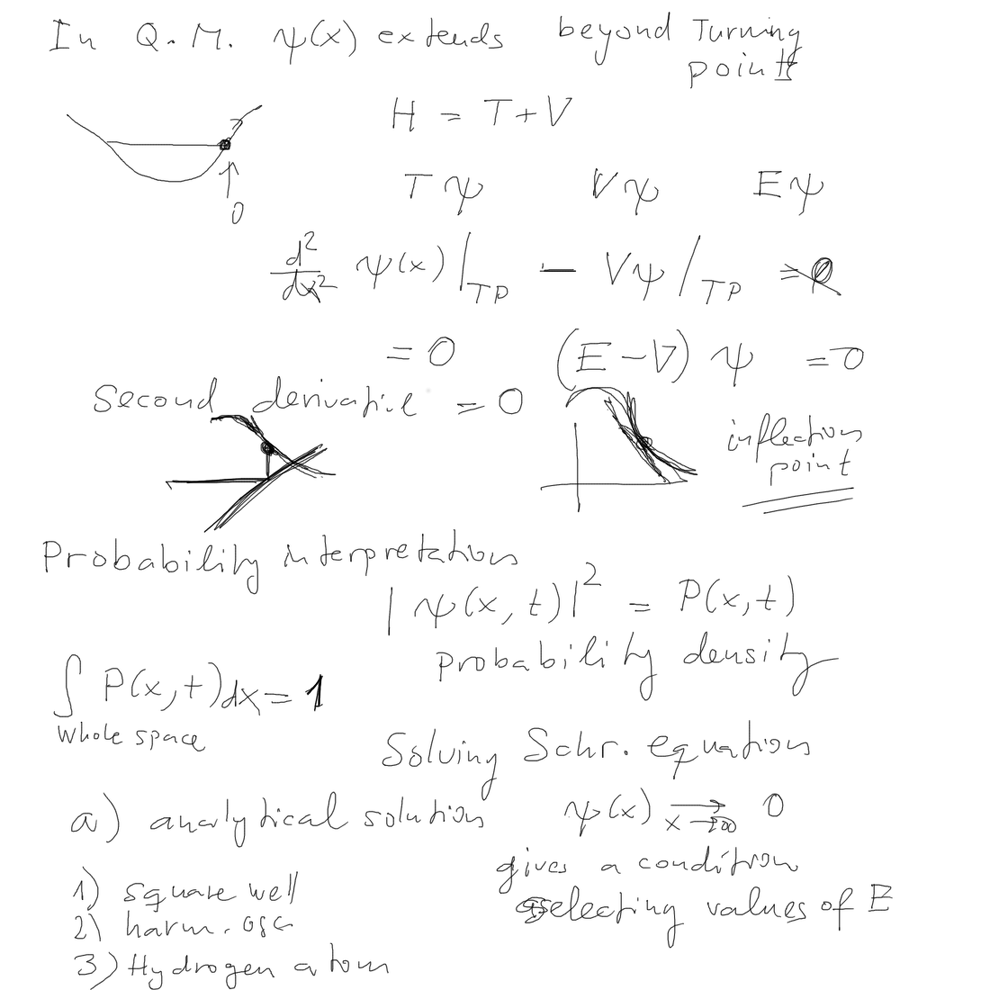
05_how_to_plot_radial_Angular_POLAR_PLOTS.png
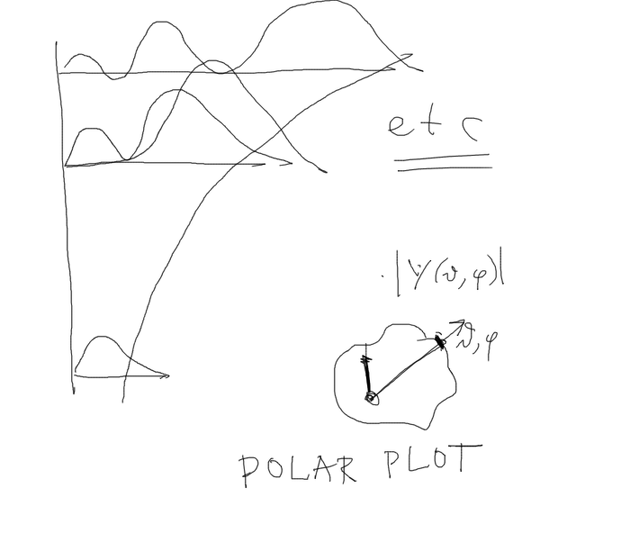 05_how_to_plot_radial_Angular_POLAR_PLOTS.png |
05_typical_plot_hydrogen.png
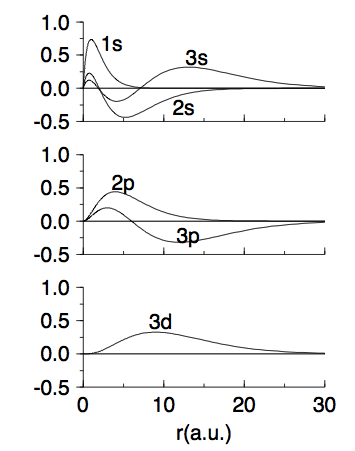 05_typical_plot_hydrogen.png |